Education Theories for A-Level Mathematics

Before analysing principles, theories, models, and numeracy, I have set out my understanding of what these mean terms actually mean:

• A theory is an idea that has been tested a number of times, and which has been found to explain ideas, (albeit they cannot usually be proven to be fully conclusive in all cases).  A model explains how things work, for example it could be a process by which people learn, or a pattern of behaviour.  Theories can be the basis for creating a model on which the theory can be tested, and verified.
• The terms theory and model are often used interchangeably, and I have noted little difference between the uses of the words in the majority of the research I have done on this subject.
• A principle is generally something which is taken to be fact, and may be used as a basis for a theory.  Throughout this essay, the terms theory and model shall be used interchangeably unless otherwise stated.
• Numeracy, sometimes called mathematical literacy is, according to the Oxford Dictionary, ‘the ability understand and work with numbers’, and in teaching it is considered to be the skills needed to function fully in modern life – for example, at work, as consumers, managing finances, making sense of health information.  According to the national numeracy organisation it means being able to:

• interpret data, charts and diagrams
• process information
• solve problems
• check answers
• understand and explain solutions
• make decisions based on logical thinking and reasoning.

This essay seeks to understand the theories which exist in education, consider any evidence of the testing of these theories, as well as alternative views, and extrapolate the theories into their potential use in the teaching of functional and ‘A’ level mathematics.

As well as being tested within a human learning environment, theories are often tested outside of the classroom.  There are many examples of animals being used to test certain theories.  In order to bring the academic theory to life for this essay, I shall, wherever possible, also test the theories being considered.  Being fortunate enough own two pet Kune Kune pigs (Mutley and Matilda), I am able to test some of the theories to try to corroborate for my own benefit the validity of the theories presented, whilst acknowledging that this testing is not under a strict testing environment!

First of all it is worth pointing out that there is a difference between an error (ie a random mistake which under ideal conditions with time to review, would not occur), and a common error or misconception (a mistaken belief).  A misconception means that the student would have got a wrong answer thinking it was correct, whereas they would spot an error given the time and ideal conditions.  Common errors or misconceptions are often seen on numerous learners’ scripts.

There is some debate about the difference between errors and misconceptions. Confrey (1990) defines both errors and misconceptions as resulting beliefs that students hold, suggesting that the misconceptions are attached to particular theoretical positions. Other researchers, such as Nesher (1987), use the term misconceptions to describe systematic errors. VanLehn (1982, in Confrey, 1990) and Brown and Burton (1978, in Dickson et al., 1984), use a further term, ‘bug’, to describe such errors.

Swan (1990) for example identified two sets of misconceptions held by students: those that affected their calculations using the four operations (addition, subtraction, multiplication and division); and those affecting their interpretation of graphs.

Changes to the content of specifications, or the way that Mathematics is taught mean there may now be previously unidentified misconceptions as a result, and it is worth bearing this in mind when trying to address any such misconceptions.

There is a lot of research in this area, and one very useful table of common errors can be found in a paper produced by a working group whose aim was to produce research material and guidance for teachers to support the planning for misconceptions.

[https://www.ncetm.org.uk/public/files/…/Misconceptions+with+the+Key+Objectives2….]

There is also useful information to be found in ‘common errors in mathematics’

[http://www.cambridgeassessment.org.uk/research-matters/]

I have added some of the detail in relation to the misconceptions from both these sources in Appendix 2, this level of detail may be useful when planning my lessons.

We now consider why misconceptions occur [Swan 2005]:

We can assess that learners make mistakes or errors for many reasons. For example:

• lapses in concentration
• hasty reasoning
• a failure to notice important features of a problem (not reading the question properly)

Others, however, are more common mistaken beliefs and could for example be due to earlier experiences in terms of learning mathematics:

_ “you can’t divide smaller numbers by larger ones”;

_ “division always makes numbers smaller”;

_ “the more digits a number has, then the larger is its value”;

_ “shapes with bigger areas have bigger perimeters”;

_ “letters represent particular numbers”;

_ “‘equals’ means ‘makes’”.

Put another way, the learner is taking knowledge of mathematics that may be correct in one situation and applying it to a different area of mathematics, where it is not correct.  These beliefs may be difficult to address since the learner sees the methodology they use working in some situations.

Bobby Ojose summarised a number of common misconceptions with reasons why they occur:

• 1/4 is larger than 1/2
  • because 4 is greater than 2
• the operation of multiplication will always increase a number
  • students’ learnt the multiplication of a positive number by a fraction less than one later in the learning process, and because of the students’ overriding need to make sense of the instruction that they receive, they had already formed the wrong belief
• adding tops and bottoms of fractions
  • the rules for adding fractions with like and unlike denominators are quite different.  Moving from adding fractions with like denominators to adding fractions with unlike denominators requires learners to make sense of the different scenarios and make adjustments –i.e. change the beliefs they have already built.
• when decimals are introduced with addition, 0.4 + 0.7 equals 1.1 (one decimal place), but with multiplication of decimals, 0.4 × 0.7 equals 0.28 (two decimal places).
  • The discrepancy from addition to multiplication with decimals could be a reason for learners to have misconceptions.

Transition often creates cognitive conflicts because the process requires unlearning what has been previously learned.

The theory behind behaviourism is that learning is the establishment of a new behaviour pattern.   Behaviourism focuses on a change in external behaviour achieved through using reinforcement, repetition, and punishment.  Reinforcement and punishment are important strategies used, and there are two types of reinforcement, positive and negative.

A summary of the meaning of these terms is:

• positive reinforcement – aims to increase good behaviour; it is a reward for behaving well, or learning a lesson
• negative reinforcement – aims to promote good behaviour; removes an unpleasant consequence
• punishment is an unpleasant consequence.

Examples of the testing of this theory was performed by Pavlov (1849 – 1936) and BF Skinner (1904 -1990), Pavlov tested his ideas on dogs, and Skinner on rats.  The results of the testing was at the time then generalised to humans.

Theories need to be tested, and some examples of the research and tests are set out below:

The Dog

Pavlov found that dogs would produce saliva when they heard or smelt food in anticipation of feeding – this is actually a normal dog behaviour!  However, Pavlov, also found that, after having played sounds to the dogs at feeding time, they also began to salivate at hearing the sounds, before any food could be seen or smelt.  The dogs effectively anticipated the food once hearing the sounds.  He also found that the dogs would begin to salivate when a door was opened for the researcher to feed them.  This response demonstrated the basic principle of classical conditioning – a non-related event, such as the playing of sounds, was associated with another event (being fed).

The Rat

Skinner found the following results from an experiment with a rat using food as a reward:

• The rat was placed in a box, and food was occasionally delivered through an automatic dispenser over the course of a few days.
• The rat approached the food tray as soon as the sound of the dispenser was heard, clearly having learned that the food would follow.
• A small horizontal section of a lever protruding from the wall had been resting in its lowest position, this was raised slightly so that when the rat touched it, it moved downward. This closed an electric circuit and operated the food dispenser. Immediately after eating the food the rat began to press the lever fairly rapidly. The behaviour has been reinforced by a single consequence.

There has however, been much criticism of the theory of behaviourism over a number of years, both in relation to its validity on training animals, and its use in teaching mathematics.

Criticisms – The Child

An example of this criticism with specific regard to the application of behaviourism in the learning of mathematics came from Stanley Erlwanger (1975), whose study showed that children could pass mastery tests to a very high level, without having any real understanding of what they were actually doing. In one test he was surprised to find a top student repeating the same mistakes over and over again.  The child had developed a totally inadequate mechanical view of the nature of mathematics through behaviour type learning, which had gone unnoticed by teachers.

At the time, this was not documented as a ‘common error’, or ‘misconception’, however it seems to me that this is exactly what this is, and its cause is due to having learnt a mechanical methodology which is incorrect in some situations.

Criticisms – The Pig

Keller Breland (1961) who is most famous for his training of marine mammals and dogs, was B.F. Skinner’s first graduate student at University of Minnesota.  Breland and Marian Breland went on to train animals for roadside tourist attractions. Using standard behaviourism techniques, they trained animals to perform complex behaviours for food reinforcement. However, in each case, after the behaviour was established, it was disrupted by instinctive behaviour used by that species to gather or prepare food.  For example, a pig was reinforced with food for dropping large wooden disks into a piggy bank. It successfully learned this task, however, it soon began dropping the coin on the way to the piggy bank, pushing it through the dirt with its nose, and flipping the coin up in the air. This is a species-typical behaviour of pigs called rooting, which prevented the pig from completing its task and receiving food reinforcement.  (I can confirm that rooting is very much a typical behaviour of pigs which in some cases is discouraged by putting a ring through the pig’s nose – note that Mutley and Matilda do not have a ring through their nose!).

The Brelands recognised that this phenomenon of instinctive drift contradicted the reinforcement theory, and soon Breland, and others with similar observations, influenced other researchers into looking at such techniques with more skeptisim.

My Test

Despite the criticism which exists with regard to the theory of behaviourism, it is still widely used.  Indeed in my own limited experiences of training 2 pigs, it appears to have worked – my husband and I have trained Mutley and Matilda to spin round, sit and reverse before receiving a treat of food.  It would be interesting to know what Breland would make of this.  Sadly I have yet to achieve such good responses from my daughter when trying to ensure she revises for her English Literature exam.

Using behaviourism in teaching functional and ‘A’ level maths

Any theory should be considered both at the planning stage and throughout, as different theories may work in different environments and for different people.

Aside from the general use of behaviourism to ensure good class behaviour (e.g. giving good marks for homework – reinforcing good behaviour, or giving detention for bad behaviour – punishment), the behaviourist approach to teaching involves repetition and the breaking down of learning tasks into smaller compact tasks.  Examples of how the theory could be put into practice could be:

• Setting clear, unambiguous objectives in terms of what will be learnt in the lesson
• Testing knowledge before and after the lesson to assess whether the student can move onto the next topic
• Offering feedback after a test (positive or negative – in the form of a grade) to condition learners to be more equipped for the real exam.
• Repetition – such as repeating the times table at the beginning of each lesson (this was the approach my junior school teacher took some decades ago – and it worked for me)

I have had some positive experience of using behaviourism in relation to teaching ‘A’ level maths.  One of my ‘A’ level students was stuck on integration by parts; I extracted all the past questions on this topic (around 15 questions).  I then explained the general approach to tackling this type of question, and asked the student to then systematically apply the approach to each question, giving guidance on any tricky areas as she went through.  Each time she got a question right she was rewarded with positive praise.  By the end of the session she was able to answer any question from the list on her own.  It is however worth considering the alternative view to this, in that the student was very early on capable of understanding the approach, and it is possible that it was more the initial explanation as to how to attempt the question, as opposed to the repetition.

One area where I do consider it to be a potential benefit is in memorising formulae (for example in trigonometry, and integration).  Unless the question asked for it, it would be impractical to derive for example the differential of ln(x) in a 5 mark question from first principles. Such formulae should be memorised, and the use of behaviourism may be a possible way of doing this.

I do not consider this to be a key method to use for teaching functional maths.  It is likely that this approach has already failed, and learners need a more engaging approach.

Cognitivism

Background

The Oxford English Dictionary defines cognition as ‘The mental action or process of acquiring knowledge and understanding through thought, experience, and the senses’.  This differs from behavioural learning in that it does not rely fully on external factors, but rather uses internal resources.

Cognitive learning theories emerged as a response to behaviourism, and began with Gestalt psychology which was developed in Germany in the early 1900s by three German theorists (Wolfgang Kohler being the original founder). The basis of the Gestalt theory is that ‘the whole is greater than the sum of its parts’; learning is more than just invoking mechanical responses from learners, i.e. it is more than behaviourism.  ‘The whole is greater than the sum of the parts’ is like looking at the whole picture rather than the constituent parts, rather like a child looking at a box of lego, and imagining the resulting house that could be made.

Other theories have evolved out of the original Gestalt learning theory, with different forms of the Gestalt theory taking shape. The field of Gestalt theories have come to be acknowledged as a cognitive-interactionist family of theories.

The Gestalt theory placed its main emphasis on cognitive processes of a higher order, causing the learner to use higher problem solving skills. They must look at the tasks presented to them and search for the underlying similarities that link them together into a cohesive whole. In this way, learners are able to determine specific relationships amongst the ideas presented.

Many psychologists have researched cognitive learning theory including, Jean Piaget, Benjamin Bloom, Jerome Bruner, David Ausubel.

Piaget constructed models of child development and identified 4 development stages:

• Sensory motor – understands the environment through basic senses
• Intuitive/pre-operational – use of memory, imagination, creativity
• Concrete operational – can go beyond the basic information but still depends on concrete material and example to support reasoning
• Formal operational – abstract reasoning becomes increasingly possible

Bloom’s taxonomy identified a hierarchical order of the cognitive processes involved in learning, which was updated and is illustrated below:

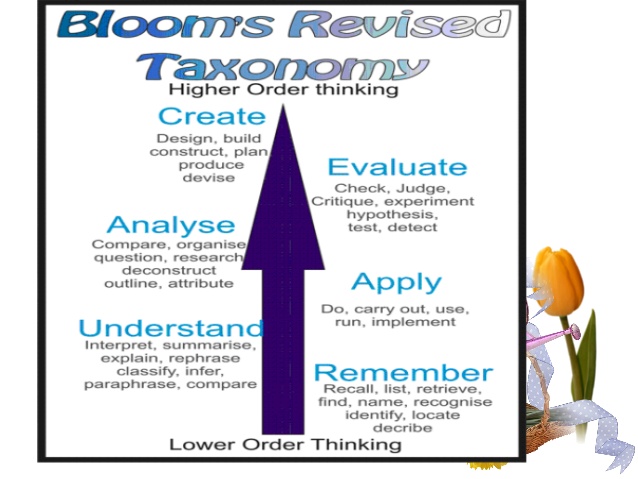
Bruner extended aspects of Piaget’s theory, and identified three ways in which learners process information, by manipulating objects, using visual images, and symbols (such an language).

Ausubel, on the other hand stressed the importance of active mental participation in meaningful learning tasks, and he made the distinction between meaningful and rote learning (learnt as isolated pieces of information).

The Chimpanzee

Wolfgang Kohler 1887 – 1967 performed extensive research using chimpanzees (one might say more than required!).

Kohler made the distinction between behaviour and intelligence. For example, we tend to speak of “intelligence” when, circumstances having blocked the obvious course, the human being or animal considers a roundabout path, to get to the goal.

Kohler’s experiments were set up in this way, the direct path to the objective—usually a banana—being blocked, but a roundabout way being left open.

One of Kohler’s examples clearly demonstrated how knowledge of the lay of the land known beforehand might be used to plan an indirect circuit through it:

The experiment was set up in a room with a very high window, with wooden shutters, that looks out on a playground. The playground could be reached from the room by a door, leading into a corridor, with a door opening on to the playground. This layout is well known to the chimpanzees. Kohler took Sultan (one of the chimpanzees) with him from another room, led him across the corridor into the room with the high window.  Kohler then opened the wooden shutter and threw a banana out, so that Sultan could see it disappear through the window, but, on account of its height, did not see it fall. Sultan immediately pushes the door open, runs down the corridor, and is then heard at the second door, and then in front of the window searching for the banana. This showed that if the lay of the land be known beforehand, the indirect circuit through it can be apprehended with ease. (Kohler, 1951, pp. 20-21)

The Dolphin

It is not always necessary to conduct experiments to assess whether cognitive learning exists in animals, everyday occurrences often give enough evidence of this theory.  For example, a recent article in Science daily news 29 March 2018 is surely evidence of learning which goes way beyond behaviourism [https://www.sciencedaily.com/releases/2018/03/180329095436.htm]:

University of Exeter researchers studied the impact of bottlenose dolphins on fisheries off northern Cyprus and said Mediterranean overfishing had created a “vicious cycle” of dolphins and fishers competing for dwindling stocks. Fishing businesses in the area are mostly small-scale, and the study says damage done by dolphins costs them thousands or even tens of thousands of euros per year. Acoustic “pingers” designed to deter dolphins were ineffective, and may even have worked as a “dinner bell” to attract them in some cases, the researchers found — though more powerful pingers might work better. “It seems that some dolphins may be actively seeking nets as a way to get food,” said lead author Robin Snape, of the Centre for Ecology and Conservation on the University of Exeter’s Penryn Campus in Cornwall. The nets examined, which are weighted to create a barrier about 1.2m high on the sea floor, are the most common kind used in the Mediterranean. When damaged by dolphins, nets may have large sections missing. The research was supported and partly funded by the Society for the Protection of Turtles.

 

The acoustic ‘pingers’ above may be considered to be close to behaviourist learning, but some thought process will have been used to determine that the nets needed to be damaged in order to release the fish.

The Child

Piaget studied his own children and the children of his colleagues in Geneva.

Piaget’s (1936) theory of cognitive development considers how a child constructs a mental model of the world. He regarded cognitive development as occurring due to biological maturation and interaction with the environment.

Piaget wanted to understand how fundamental concepts like the very idea of number, time, quantity, causality, justice and so on emerged.

Piaget showed that rather than children being simply less competent thinkers than adults, they actually think in very different ways compared to adults.

Piaget, believed that children are born with a very basic mental structure (genetically inherited and evolved) on which all subsequent learning and knowledge are based.  He did not consider that a particular stage was reached at a certain age – however an indication of the age at which the average child would reach each stage is usually shown:

Sensorimotor Stage (Birth-2 yrs)

Knowing that an object still exists, even if it is hidden.  This requires the ability to form a mental representation (i.e., a schema) of the object.

Preoperational Stage (2-7 years)

This is the ability to make one thing – a word or an object – stand for something other than itself.

Concrete Operational Stage (7-11 years)

This marks the beginning of logical or operational thought.  The child can work things out internally in their head (rather than physically try things out in the real world).

Children can conserve number, mass, and weight. Conservation is the understanding that something stays the same in quantity even though its appearance changes.

Formal Operational Stage (11 years and over)

During this time, people develop the ability to think about abstract concepts, and logically test hypotheses.

Criticisms – The Child

Some criticisms of Piagets’s work were:

• The sample was very small;
• The sample it was composed solely of European children from families of high socio-economic status.

Dasen (1994) cites studies he conducted in remote parts of the central Australian desert with 8-14 year old Aborigines. He gave them conservation of liquid tasks and spatial awareness tasks. He found that the ability to conserve came later in the aboriginal children, between aged 10 and 13 (as opposed to between 5 and 7, with Piaget’s Swiss sample).

He also found that spatial awareness abilities developed earlier amongst the Aboriginal children than the Swiss children. This demonstrates that cognitive development is not purely dependent on maturation but on cultural factors too.

My Test

I can confirm that Mutley and Matilda have both found interesting ways of escaping their enclosure to root further afield.  One involved Mutley lifting a heavy gate on its hinges, and Matilda pushing it open after the latch lifted.

Using cognitivism in teaching functional and ‘A’ level maths

Any theory should be considered both at the planning stage and throughout, as different theories may work in different environments and for different people.

The various different theories could lead to slightly different practical implementation.

For learning generally, cognitive theory implies that learning is cumulative, and planning should take both this and the fact that the learning should be age appropriate into account.  This means that the learning should be sequenced to build on what has previously been learned.

The learning plan should allow students to be actively involved in meaningful tasks, and interact with the curriculum material, meaning a lot of organisation is required.

For A level maths, for example, this would mean setting an order to the learning to ensure that each piece of learning is built upon, and that new topics are taught in order.

For entry level numeracy, the learning needs to be made very meaningful to the students, for example linking the learning to everyday events in the students’ lives, such as shopping at the greengrocers, or calculating the time required to cook a turkey.

Information processing theory

Background

The Oxford quick reference dictionary defines information processing theory as

‘A theory that views humans as information processing systems, which take in information from the environment, process it, and then output information to the environment in the form of movement. The theory is based on the proposition that humans process the information they receive, rather than merely responding to stimuli. Many cognitive processes are involved between the reception of a stimulus and the response of the individual, these include stimulus identification, storage and retrieval of information, perception, and decision making’.

[https://psychologenie.com/information-processing-theory]

This theory compares processing of information by humans to those of computers. In 1956, American psychologist George A. Miller believed that the mind receives the stimulus, processes it, stores it, locates it, and then responds to it. He also stated that the human mind can only hold 5-9 chunks of information at a time.

Miller said that learning is simply a change in the knowledge that has been stored by the memory. There is a fixed pattern of events that take place in learning, and by knowing this pattern we can enable children and adults with special abilities to learn new things faster.
The following diagram describes the information processing model in detail.

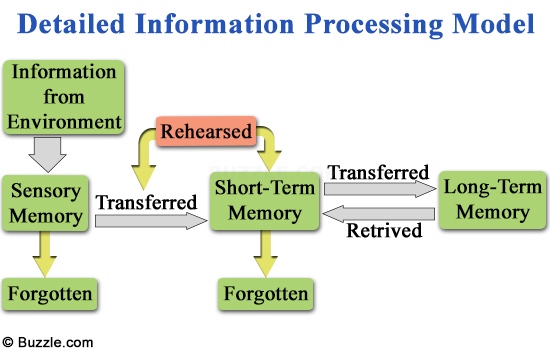
In order to process information, we need to:

• focus our attention (and hold it),
• use our perception (interpret incoming information),
• memorise the information (the filing of the information for future use).

There are many different theories of information processing that focus on different aspects of perceiving, remembering, and reasoning. However, one thing that they agree on is that elaboration is a key to permanently storing information for its quick retrieval.

Neuro-Cognitive Approaches

It is notable that there is little in the way of research that has been performed to test information processing theories. Over time, various methods of examining the brain have evolved, and early theories have already been superseded by newer theories.  The most recent views are termed ‘whole-brain theory’, which means that all of the brain is involved in most activities.  Learning is the result of the formation or neural pathways (the basic components of the brain – neurons – making connections).  It is thought that the number of connections is not endless, and as such old or weak connections get destroyed as new connections are made.

Although in its infancy in terms of research, neuroscience appears to be broadly agreeing with the conclusions of information processing theory, whilst adding that keeping the brain in the best condition is key (e.g. ensuring adequate supply of food and water).

Criticisms

Given the lack of research, it is difficult to find any tested evidence which can be used to criticise information processing theory.  However obvious things to consider would be that people’s brains may work differently to other people’s brains, and if this were not the case, everyone would learn at the same speed and to the same ability (given the same learning plans).  External environment and emotions must surely also play a part in the ability to learn.

Using information processing theory in teaching functional and ‘A’ level maths

Any theory should be considered both at the planning stage and throughout, as different theories may work in different environments and for different people.

For learning generally, information processing theory means that in the first instance, you need to gain the attention of the students so that the brain has something to process.  If this is not done, then there is little point in continuing.  The use of underlining, and colours to attract the attention can help to gain attention, as well as asking questions at the start of the lesson.

The next step is to maintain that attention, and this depends on a person’s attention span.  Having active engagement helps to maintain the attention for longer, having more but shorter activities also helps.  Allowing group work is also useful for maintaining attention.

Perception – in order to help students to understand and process the information they are taking in, the following steps should be taken:

• the teacher needs to explain how the new information links to the previous information;
• the information needs to be given context – use examples;
• encourage ideas from students.

Memory – by presenting information in a structured manner, and summarising the key points, the learners should be able to memorise the information.  Recapping in future lessons will also help.  The use of visuals will also help students to memorise information.

For entry level numeracy, again, the learning needs to be made personal for students.  Lots of interaction and discussions on when topics may be relevant to their every-day lives, for example when checking change in the corner shop.

Social Learning Theory

Background

The basis of social learning theory is that people learn from other people, and generations learn from previous generations.  We do not however ‘mindlessly’ adopt the behaviours of others, rather we make decisions as to what examples to follow.  As such, Bandura (1977), one of the earliest social learning theorists renamed the theory to be social cognitive theory.

[https://www.learning-theories.com/vygotskys-social-learning-theory.html Lev Vygotsky (1896-1934)]

The ‘More Knowledgeable Other’ (MKO) refers to anyone who has a better understanding or a higher ability level than the learner (e.g. a teacher, coach, or older adult).

MKOs give support in terms of ‘scaffolding’ (Bruner) – support is given during learning and taken away when no longer required.  Scaffolding can take a number of forms:

• explanations, guidance, practice
• giving clues and feedback
• structuring tasks

The Bobo Doll

[https://www.simplypsychology.org/bobo-doll.html]

Bandura (1961) conducted a study to investigate whether social behaviours (i.e., aggression) can be acquired by observation and imitation. Children were individually taken into a room containing toys and they played pictures for 10 minutes.  Then they were split into three groups:

1. 24 children (12 boys and 12 girls) watched adults behaving aggressively towards a toy called a ‘Bobo doll’. The adults attacked the Bobo doll using a hammer in some cases, and in others threw the doll in the air and shouted “Pow, Boom.”
2. 24 children (12 boys and 12 girls) were exposed to a non-aggressive model who played in a quiet and subdued manner for 10 minutes (playing with a tinker toy set and ignoring the bobo-doll).
3. 24 children (12 boys and 12 girls) were used as a control group and not exposed to any model at all.

Each child was (separately) taken to a room with relatively attractive toys, but as soon as the child started to play with the toys, the experimenter told the child that these were the very best toys were reserved for the other children.

The next room contained some aggressive toys and some non-aggressive toys. The non-aggressive toys included a tea set, crayons, three bears and plastic farm animals. The aggressive toys included a mallet and peg board, dart guns, and a 3 foot Bobo doll.

Children who observed the aggressive model made more aggressive responses than those who were in the non-aggressive or control groups.

The girls in the aggressive model condition also showed more physical aggressive responses if the model was male, but more verbal aggressive responses if the model was female. However, the exception to this general pattern was the observation of how often they punched Bobo, and in this case the effects of gender were reversed.

Boys were more likely to imitate same-sex models than girls. The evidence for girls imitating same-sex models is not strong.

The findings support Bandura’s (1977) Social Learning Theory. That is, children learn social behaviour such as aggression through observation learning.

The Pigs

[https://phys.org/news/2017-10-kune-piglets-social-skills-astonishingly.html]

A study with Kune Kune pigs conducted by cognitive researchers from the Messerli Research Institute of Vetmeduni Vienna, evidences that they do in fact learn from each other – in this example from their mother or their aunt. They also possess a remarkable long-term memory once they have internalised a technique. The results were published in the journal Animal Behaviour.

The researchers showed that free-ranging Kune Kune piglets attentively observed and replicated tasks demonstrated by their mother or an aunt.

The objective of the study was to demonstrate social learning through the passing on of knowledge to the next generation. (Most previous studies looked at animals learned from peers). The task involved opening the sliding door of a food box to get at a piece of food. The animals could use their snout to move the door to the left or right into one of three positions: left, right or middle.

Three groups of six piglets were used. Two observed their mother or aunt as they used one of two possible opening techniques. The third control group had to figure out the task without observation.

The findings from the trials showed that the control group used all possible techniques, confirming that that there was no predisposition or bias. The observer piglets, on the other hand, exhibited true learning through observation, they either copied the push direction or both direction and position together. Notably, the piglets produced the best results when they were tested the next day. Apparently, they memorised what they observed and could correctly reproduce it when needed.

Interestingly, the control group remembered a particular solution if they found one after a few attempts. The piglets could also immediately recall the same solution 6 months later.

The researchers believe that social learning among Kune Kune pigs is related to the fact that the pigs live in natural family groups under free-ranging conditions, triggering an existing aptitude for social intelligence among these animals.

If such a test were to be performed on pigs in very different conditions, a different outcome would give weight to the idea that the external environment also make a difference to a learning outcome.

Biological Evidence

[https://www.ncbi.nlm.nih.gov/pmc/articles/PMC3510904/]

‘One of the biggest neuroscience discoveries in the last decade was mirror neurons.  These neurons respond to actions that we observe in others in the same way as they do when we actually recreate that action ourselves. This gives biological evidence for social learning, and defects in the mirror neuron system are being linked to disorders like autism. ‘

Criticisms

The Bobo doll test involves the child and an adult model, with no interaction between the child and the model at any point.  Also, the model and the child are strangers. This, is quite unlike a ‘normal’ situation, which often takes place within the family.

Cumberbatch (1990) believes that there was a ‘novelty’ value effect in the bobo doll test.  He found that children who had not played with a Bobo Doll before were five times as likely to imitate the aggressive behaviour than those who were familiar with it.

A further criticism of the study is that the demonstrations are measured almost immediately. With such snap shot studies, we cannot discover if such a single exposure can have long-term effects. Note however that the Kune Kune study did show long term effects.

https://www.simplypsychology.org/bandura.html

‘Social learning theory cannot explain the whole range of behaviour we develop, including thoughts and feelings.  This is why Bandura modified his theory and in 1986 renamed his Social Learning Theory, Social Cognitive Theory, as this better described how we learn from social experiences. ‘

Using social learning theory in teaching functional and ‘A’ level maths

Any theory should be considered both at the planning stage and throughout, as different theories may work in different environments and for different people.

Generally in the classroom, scaffolding can be used in a number of forms, for example:

• Through explanations, guidance and practice
• Interacting and asking questions
• Holding workshops to learn from peers.

Specifically in relation to entry level mathematics, it can help to engage actively and encourage buddying and testing each-other’s work.

Humanism

Background

This theory states that humans strive to maximise their personal growth to reach their full potential.  However Maslow (1968) also recognised that barriers existed which prevented people reaching their full potential, and these had to be addressed before the full learning potential could start.

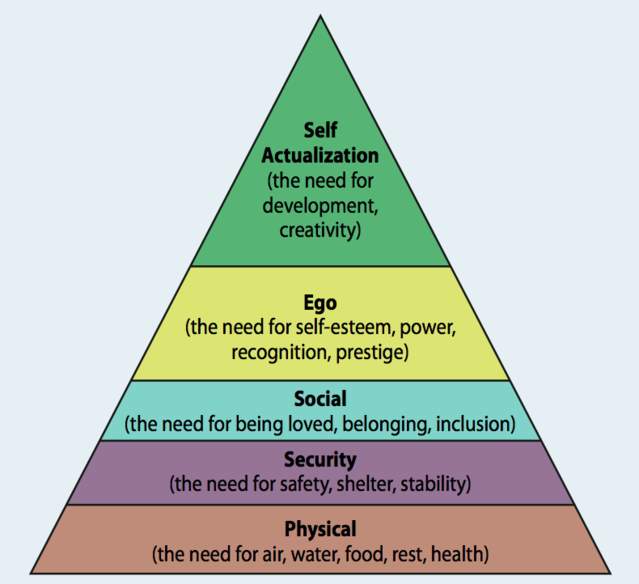
Maslow’s heierarchy of needs.

[https://www.psychologytoday.com/us/blog/hide-and-seek/201205/our-hierarchy-needs]

Rogers (1989) was responsible at the time for introducing the term ‘facilitation’ into use within education. In other words, teachers should be more interested in the process of learning as opposed to the content, and the learners should take control of their own learning,

Criticisms

A criticism is that this theory is philosophical as opposed to scientific, and, as such harder to prove through tests.

Using humanism theory in teaching functional and ‘A’ level maths

Any theory should be considered both at the planning stage and throughout, as different theories may work in different environments and for different people.

In general, the learning environment needs to be safe and comfortable.  Thereafter teachers should be able to facilitate lessons and help learners come to their own conclusions.

This theory may be relevant to adults at entry stage numeracy.  It could be that tapping into the barriers that exist may help adults to get over the initial hurdles.

Andragogy

Background

Andragogy refers to a theory of adult learning, and is based on the theory that adults learn differently to children. For example, adults are likely to have chosen to learn (as opposed being sent to school), are more self-directed, internally motivated, and ready to learn.

Malcom Shepherd Knowles (1913-1997)

Knowles set out four assumptions to consider when teaching adults.

1. Self-concept – Adults are self-directed, and should input into the content and process of their learning.
2. Experience – with age comes much experience to draw from, their learning should focus on adding to what they have already learned in the past.
3. Readiness to learn – Content should focus on issues related to their work or personal life, otherwise there is no reason to learn.
4. Orientation to learning – learning should be centred on solving problems instead of memorising content.

Two further assumptions were published later:

5. Motivation – not just in terms of a better salary, but also self-esteem.
6. Need to know – meaning how important is it to learn a particular topic.

Criticisms

This is another untested theory.  Knowles himself said his theory did not apply to all adults, and in his later work, he emphasized how each situation should be assessed on an individual basis to determine how much self-direction would be helpful for students.

Using andragogy theory in teaching functional and ‘A’ level maths

Any theory should be considered both at the planning stage and throughout, as different theories may work in different environments and for different people.

For general learning, including A level maths, this theory implies that online learning can benefit from Knowle’s theories of self-directive learning.  So for example giving homework using the internet bitesize material for example could work well.

For entry level numeracy, lessons can be more effective by actively engaging adult students. For example, use of small group discussions, and ensuring that the content builds on experience.

Modern Practice

[http://www.educationaldesigner.org/ed/volume1/issue1/article3/]

improving_learning_in_mathematicsi (1).pdf

 

Background

Today, there are a number of papers which, rather than focus on one learning theory, focus on designing practical tools to use in the classroom.  Malcolm Swan is one well known example of someone is proven to have helped many students with his approach.

Some direct quotes from the tes obituary 30 May 2017 succinctly sums up the contribution made by Malcolm Swan to learning theories in mathematics:

‘Ask any group of maths teachers about the strongest influence on their work and career and very many will name Professor Malcolm Swan, who has died at the age of 64. Those who have met him will remember his warmth, passion and creativity, but all who have been touched by his work will feel the influence he has had on their thinking and their very nature as teachers.

The impact of the rich resources created by Malcolm and his colleagues at the University of Nottingham’s Shell Centre has been truly transformative, changing the thinking of mathematics teaching and improving the mathematical learning experience of countless students.

In a subject where lessons were largely teacher-led and involved a deal of demonstration of technique followed by exercises to practise the same skills, a lesson based on Malcolm’s work was for many a revelation: students, often working in groups, discussing mathematical points and arguing over a solution – sometimes passionately! – with the emphasis always on prompting students to do the thinking. Getting students to stop working at the end of a lesson became a frequent but welcome headache for teachers.

Malcolm’s exceptional skill was in the design of tools that enable teachers of mathematics to turn research insights into happy learning in their classrooms. He did this through a combination of a deep understanding of the mathematics and the learning process, creative ideas and a genius for design.

His lessons contain surprise and delight, humanity and humour: qualities not always associated with mathematics classrooms, but ones that helped students’ understanding, particularly the many who struggle with the subject.

Amongst his early projects, Malcolm designed The Language of Functions and Graphs to help teachers prepare their students for this new exam topic. Its quality was recognised years later when he was awarded the International Society for Design and Development in Education’s prize for excellence in design.

For the last 25 years, international collaborations widened the impact of the Shell Centre’s work. In a recent US-based project, Malcolm led the design of 100 lessons that took forward teaching on concept development and on problem solving. There have been more than 7,000,000 lesson downloads so far from the Mathematics Assessment Project – an impact reflected in teacher enthusiasm in the Twittersphere and beyond.

In 2015, the work was recognised by a new award of the International Commission for Mathematical Instruction “for more than 35 years of development and implementation of innovative, influential work in the practice of mathematics education…”. ‘

Professor Malcolm Swan has shown that the most effective way to develop mathematical thinking skills is to teach using active and collaborative methods.  He has set out 8 principles for teaching maths:

(i) Build on the knowledge learners bring to sessions:

Teaching is more effective when it assesses and uses prior learning to adapt to the needs of learners.

(ii) Expose and discuss common misconceptions:

Conflicts, when resolved through reflective discussion, mean learning will be remembered more than when using conventional, incremental teaching methods, which avoid learners making ‘mistakes’.

(iii) Develop effective questioning:

A variety of lower-level and higher-level open questions is much more beneficial than a continuous diet of closed recall questions.

Also, it is important to allow time for learners to think before offering help or moving on to ask a second learner. Studies have shown that many teachers wait for less than one second, which is not enough.

(iv) Use cooperative small group work:

It is essential that a supportive and encouraging atmosphere is created in the learning environment.

(v) Emphasise methods rather than answers:

Learners often see their task as ‘getting through’ an exercise rather than working on an idea. Using this method, learners may work on fewer problems than in conventional texts, but they come to understand them more deeply as they tackle them using more than one method.

(vi) Use rich collaborative tasks:

Rich tasks:

_ allow learners to make decisions;

_ involve learners in testing, proving, explaining, reflecting, interpreting;

_ promote discussion and communication;

_ encourage originality and invention;

_ encourage ‘what if?’ and ‘what if not?’ questions;

_ are enjoyable and contain the opportunity for surprise.

Simple tasks do not motivate a need to learn.

(vii) Create connections between mathematical topics:

Many things learned in one topic are used in others, making this linkage helps the learner to maintain their understanding.

 

(viii) Use technology in appropriate ways

Technology offers the opportunity to present mathematical concepts in dynamic, visually exciting ways that engage and motivate learners.

Bobby Ojose (2015) also recommended some practical ways to deal with common errors in mathematics.  These are shown in the section below.

Uses in teaching functional and ‘A’ level maths

For A level maths, Malcolm Swan’s theory works well when allowing groups to work together to solve complex questions using different approaches.

For functional level maths, making the subject matter come to life is key.  Teaching topics which may involve the use of maths at various stages could help.

Take for example the topic of food.  Maths can be used in a variety of ways, with groups working together, for example:

• Measuring and ratioing ingredients to cook
• Calorie counting
• Paying for ingredients, and getting change
• Measuring the amount of sugar eaten in a day versus the recommended daily allowance.

All of these things can be related to every-day life.  Field trips could be incorporated (e.g. a trip to the café, with a view to having a snack and listing the ingredients to copy a menu).

Teaching also needs to deal with common errors and misconceptions.  When teaching functional maths to mature adults, we will be possibly dealing with some very ingrained beliefs.

There are two common ways of reacting to these misconceptions.

• Aim to prevent them developing by warning leaners as you teach
• Use the misconceptions as learning opportunities, in other words you learn from making mistakes.

In my view, the first of these approaches is unlikely to work when teaching functional maths as it is likely to be too late.  In addition research suggests that teaching approaches which focus on discussing misconceptions result in longer term learning than approaches which try to avoid

mistakes by teaching the ‘right way’.

Bobby Ojose summarised a number of ways for teachers to deal with common misconceptions.  Premature attention to rules for computation should be discouraged since the rules do not help learners think about the operations and what they mean.

The following strategies are suggested:

1. Begin with simple contextual tasks,

2. Connect the meaning of fraction computation with whole number computation,

3. Let estimation and informal methods play a big role in the development of strategies, and

4. Explore using a variety of models and have learners defend their solutions using models.

Misconception 1: Addition of Fractions

One example of models which could be used to teach operations with fractions would be the use of physical objects, like chips, to illustrate the concept of fractions with different denominators. An array form could be constructed, to show that

3/5 = 6/10 and 1/2 = 5/10. Then, that 3/5 – 1/2 = 6/10 – 5/10 = 1/10.

Misconception 2: Addition of Exponents

For example 3^4+3^4=3^8

Here, the learner thinks that they can add the powers because the base is the same for both terms.

The teacher should distinguish between 3^4+3^4 and 3^4*3^4

This could reveal the learner misconception. The use of a graphic organizer to illustrate the concept is also suggested, for example:

They should be then shown how this is different to y.y.y.y  .  y.y.y.y

Graphic organizers appear to be beneficial as an instructional strategy that aid in the retention of learned information. Many learners benefit from a visual approach to brainstorming or organizing information.  Students with learning disabilities have difficulties recalling key information, making connections between broad concepts and detail, and solving mathematical word problems. The use of graphic organizers, may be a viable strategy to help students with learning disabilities.

Researchers (e.g. Ausubel) have noted that graphic organizers aid comprehension for several reasons:

• They match the mind and, because it arranges information in a visual pattern it makes it possible for information to be easily learned and understood;
• They demonstrate how concepts are linked to prior knowledge to aid comprehension;
• They aid the memory as opposed to recalling key points from an extended test;
• They help the learner retain information readily when higher thought processes are involved; and
• They engage the learner with a combination of the spoken word with printed text and diagrams.